Türkçe Bilgi logo TürkçeBilgi

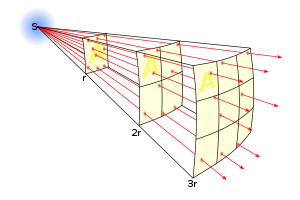
Ters Kare Yasası

Kısaca: Fizikte, ters kare kanunu belirli bir fiziksel miktar veya şiddeti o fiziksel büyüklüğün kaynağından uzaklığın karesiyle ters orantı olduğunu belirten herhangi bir fiziksel kanundur. ...devamı ☟

Ters kare yasası
Ters Kare Yasası

}

Areas of application

In particular the inverse-square law applies in the following cases: doubling the distance between the light and the subject results in one quarter of the light hitting the subject.

Gravitation

Gravitation refers to the attraction between two objects with mass. This law states:
The gravitation attraction force between two 'point masses' is directly proportional to the product of their masses and inversely proportional to the square of their separation distance. The force is always attractive and acts along the line joining them.
If we want to calculate the attraction between massive bodies, we need to add all the point-point attraction forces vectorially and the net attraction might not be exact inverse square. However, if the separation between the massive bodies is much larger compared to their sizes, then to a good approximation, it is reasonable to treat the masses as point mass while calculating the gravitational force.
This law was first suggested by Ismael Bullialdus but put on a firm basis by Isaac Newton after Robert Hooke proposed the idea in a letter to Newton. Hooke later accused Newton of plagiarism.

Electrostatics

The force of attraction or repulsion between two electrically charged particles, in addition to being directly proportional to the product of the electric charges, is inversely proportional to the square of the distance between them; this is known as Coulomb's law. The deviation of the exponent from 2 is less than one part in 1015.} This implies a limit on the photon rest mass.

Light and other electromagnetic radiation

The intensity (or illuminance or irradiance) of light or other linear waves radiating from a point source (energy per unit of area perpendicular to the source) is inversely proportional to the square of the distance from the source; so an object (of the same size) twice as far away, receives only ¼ the energy (in the same time period). More generally, the irradiance, i.e., the intensity (or power per unit area in the direction of propagation), of a spherical wavefront varies inversely with the square of the distance from the source (assuming there are no losses caused by absorption or scattering). For example, the intensity of radiation from the Sun is 9140 watts per square meter at the distance of Mercury (0.387AU); but only 1370 watts per square meter at the distance of Earth (1AU)—a threefold increase in distance results in a ninefold decrease in intensity of radiation. Photographers and theatrical lighting professionals use the inverse-square law to determine optimal location of the light source for proper illumination of the subject. The fractional reduction in electromagnetic fluence (Φ) for indirectly ionizing radiation with increasing distance from a point source can be calculated using the inverse-square law. Since emissions from a point source have radial directions, they intercept at a perpendicular incidence. The area of such a shell is 4Ï€r2 where r is the radial distance from the center. The law is particularly important in diagnostic radiography and radiotherapy treatment planing, though this proportionality does not hold in practical situations unless source dimensions are much smaller than the distance r.

Acoustics

The inverse-square law is used in acoustics in measuring the sound intensity at a given distance from the source. Inverse-Square law for sound == Examples Electromagnetic radiation Let the total power radiated from a point source, e.g., an omnidirectional isotropic antenna, be P \ . At large distances from the source (compared to the size of the source), this power is distributed over larger and larger spherical surfaces as the distance from the source increases. Since the surface area of a sphere of radius r \ is A = 4 \pi r^2 \ , then intensity I \ of radiation at distance r \ is : I = \frac = \frac. \, : I \propto \frac \, :\frac = \frac^2}^2} \, : I_1 = I_ \cdot ^2} \cdot \frac^2} \, The energy or intensity decreases by a factor of ¼ as the distance r is doubled, or measured in dB it would decrease by 6.02 dB. This is the fundamental reason why intensity of radiation, whether it is electromagnetic or acoustic radiation, follows the inverse-square behaviour, at least in the ideal 3 dimensional context (propagation in 2 dimensions would follow a just an inverse-proportional distance behaviour and propagation in one dimension, the plane wave, remains constant in amplitude even as distance from the source changes).

Acoustics

In acoustics, the sound pressure of a spherical wavefront radiating from a point source decreases by 50% as the distance r is doubled, or measured in dB it decreases by 6.02 dB. The behaviour is not inverse-square, but is inverse-proportional: : p \propto \frac \, : \frac = \frac \, : p_1 = p_2 \cdot r_2 \cdot \frac \, However the same is also true for the component of particle velocity v \, that is in-phase to the instantaneous sound pressure p \,. : v \propto \frac \, Only in the near field the quadrature component of the particle velocity is 90° out of phase with the sound pressure and thus does not contribute to the time-averaged energy or the intensity of the sound. This quadrature component happens to be inverse-square. The sound intensity is the product of the RMS sound pressure and the RMS particle velocity (the in-phase component), both which are inverse-proportional, so the intensity follows an inverse-square behaviour as is also indicated above: : I = p \cdot v \propto \frac. \, The inverse-square law pertained to sound intensity. Because sound pressures are more accessible to us, the same law can be called the "inverse-distance law".

Kaynakça

}

Kaynaklar

Vikipedi

Bu konuda henüz görüş yok.
Görüş/mesaj gerekli.
Markdown kullanılabilir.

Gauss yasası
3 yıl önce

eşdeğerdir. Aynı şekilde Newton'un kütleçekim yasası bir ters kare yasasıdır ve Gauss'un yerçekimi yasası ile eşdeğer bir niteliğe sahiptir. Yasa tümlev...

Gauss yasası, Carl Friedrich Gauss, Coulomb yasası, Direnç (elektrik), Elektrik, Elektrik akımı, Elektriksel alan, Elektriksel gerilim, Elektriksel iletkenlik, Elektriksel yük, Elektromanyetizm
Coulomb Kanunu
3 yıl önce

Coulomb yasası, ya da Coulomb’un ters kare yasası, bir fizik yasasıdır. Elektrik yüklü tanecikler arasındaki elektrostatiği tanımlar. Bu yasa 1785'te Fransız...
Manyetizma için Gauss yasası
3 yıl önce

Manyetizma için Gauss yasası, Maxwell'in klasik elektromanyetizmayı açıklayan dört denkleminden biridir. Bu yasa kapalı bir yüzeyden geçen net manyetik...
Ohm Kanunu
3 yıl önce

sebep olarak dirençte değişiklik oluştururlar. Ohm yasası Kirçoh gerilim yasası (KVL) ve Kirşof akım yasası (KCL) nu elde etmek için yeterlidir. İlk eşitliğin...

Ohm Kanunu, Amper, Direnç, Ohm, Volt
Elektrik alanı
3 yıl önce

benzerlikler: İkisi de boşlukta yayılır. İkisi de merkezidir ve korunur. İkisi de ters kare yasasına uyar. İkisi de ışık hızıyla yayılır. Elektrik yükü ve relativistik...

Elektriksel alan, Coulomb yasası, Direnç (elektrik), Elektrik, Elektrik akımı, Elektriksel gerilim, Elektriksel iletkenlik, Elektriksel yük, Elektromanyetizm, Elektromıknatıssal ışınım, Elektronik devre
İstatistiksel terimler, kavramlar ve konular listesi
3 yıl önce

karakteristik tipik olmayan özellik T-kare sınaması T-kare testi Tobit modeli Tong eşitsizliği toplam beklenti yasası toplam belirleme katsayısı toplam belirtme...
Optik
3 yıl önce

yansımayı, iğne deliği kameranın çalışma prensibini, ışığın yoğunluğunun ters kare yasası ile ilişkisini, Ay ve Güneş tutulmasını, ıraklık açısını açıklamıştır...

Optik, Fizik, Kırınım, Snell Yasası, Uzay, Geometrik optik
Henry Cavendish
3 yıl önce

arasındaki kuvvetin, aralarındaki uzaklığın karesiyle ters orantılı olduğunu ortaya attı. Elektrostatiğin bu temel yasası daha sonra Fransız fizikçi C.A. Coulomb...